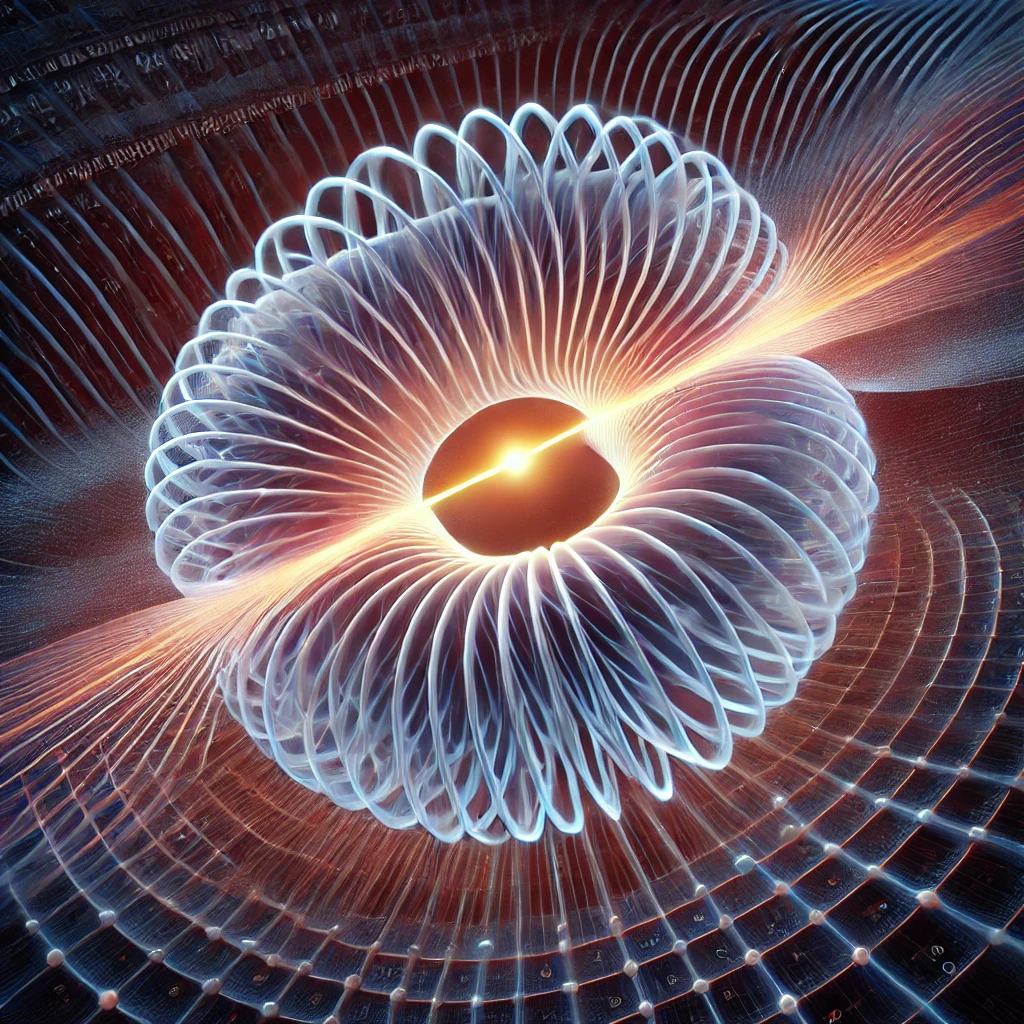
Abstract
This paper introduces a novel atomic model—the torus atom—which redefines the fundamental structure of matter. Unlike traditional spherical or orbital models, the torus atom proposes a dynamic, directional flow of energy, offering solutions to longstanding mysteries in quantum mechanics and particle physics. This model explains phenomena such as the double-slit experiment, wave-particle duality, and matter-antimatter asymmetry. Moreover, it challenges the existence of bosons as particles, reframing them as energy releases from toroidal tears, which occur during atomic interactions or collisions. Mathematical frameworks and calculations are provided to support this hypothesis, along with predictions for experimental validation.
1. Introduction
The atomic structure has undergone several transformations throughout history, from the indivisible atom of Democritus to the quantum mechanical orbitals of modern physics. While current models adequately explain many phenomena, significant gaps remain. The double-slit experiment, wave-particle duality, and the asymmetry between matter and antimatter are examples of such unresolved mysteries. This paper proposes a new atomic model based on a toroidal structure to address these gaps.
2. The Torus Atomic Model
2.1 Structure and Energy Dynamics
The torus atomic model envisions the atom as a toroidal (donut-shaped) structure, characterized by continuous energy flow along its surface. This flow is directional, creating intrinsic properties such as spin, magnetism, and chirality. The energy dynamics of the torus can be described by: Etorus=∮surfaceF⃗⋅dA⃗,E_{torus} = \oint_{\text{surface}} \vec{F} \cdot d\vec{A},
where F⃗\vec{F} represents the energy flow vector, and dA⃗d\vec{A} is the infinitesimal surface area element.
2.2 Directionality and Chirality
Directionality is a cornerstone of the torus atom, differentiating matter from antimatter. The right-hand rule governs energy flow in matter, while the left-hand rule applies to antimatter. This symmetry aligns with CPT invariance in physics: F⃗matter=−F⃗antimatter.\vec{F}_{matter} = -\vec{F}_{antimatter}.
3. Addressing the Double-Slit Experiment
3.1 Light as Escaping Energy
In the torus model, light is not a wave or a particle but energy escaping from the atom’s toroidal structure. This escaping energy exhibits both wave-like and particle-like properties depending on observation. The probability distribution in the double-slit experiment can be modeled as: P(x)=∣ψ(x)∣2,P(x) = \left| \psi(x) \right|^2,
where ψ(x)\psi(x) is the wavefunction derived from the toroidal energy distribution.
3.2 Explaining Interference Patterns
The interference pattern arises naturally from the directional emission of energy. When energy escapes, it follows toroidal paths, creating constructive and destructive interference. This can be described mathematically as: I(x)=I0cos2(kx2),I(x) = I_0 \cos^2\left(\frac{kx}{2}\right),
where I0I_0 is the initial intensity, and kk is the wave number.
3.3 Energy Fields and Path of Least Resistance
Photons, as escaping energy, travel along paths of least resistance through the surrounding energy fields. The paper or material with the slit, composed of energy atoms, acts as a dampener and redirects photons based on the cumulative average of opposing energy fields. This randomness in photon paths, combined with the energy interactions of the material, produces the observed diffraction pattern. The average paths are shaped by: P⃗path=∫F⃗env dV∫dV,\vec{P}_{path} = \frac{\int \vec{F}_{env} \, dV}{\int dV},
where F⃗env\vec{F}_{env} represents the environmental energy fields influencing the photon’s trajectory.
4. Matter-Antimatter Asymmetry
4.1 Directional Flows
Matter and antimatter differ in the direction of their toroidal energy flows. The torus model predicts that these flows create opposing magnetic moments: μmatter=−μantimatter.\mu_{matter} = -\mu_{antimatter}.
4.2 Annihilation and Energy Release
When matter and antimatter collide, their opposing energy flows annihilate, releasing pure energy. The energy released during annihilation is proportional to the overlap of their toroidal structures: Erelease=∫overlapF⃗matter⋅F⃗antimatter dV.E_{release} = \int_{\text{overlap}} \vec{F}_{matter} \cdot \vec{F}_{antimatter} \, dV.
4.3 Explaining the Right- and Left-Hand Rules
The right-hand rule in electromagnetism is a direct consequence of the toroidal energy flow in matter. For antimatter, the reversed energy flow aligns with the left-hand rule. These opposing directionalities inherently explain the symmetrical yet opposite behaviors of matter and antimatter under electromagnetic forces: F⃗matter×B⃗=−(F⃗antimatter×B⃗).\vec{F}_{matter} \times \vec{B} = -\left(\vec{F}_{antimatter} \times \vec{B}\right).
5. Reinterpreting Bosons
5.1 Bosons as Energy Releases
In the torus model, bosons are not particles but manifestations of energy released when toroidal structures tear during interactions or collisions. The randomness of the tear resembles tearing paper, where the split tends toward an average but is inherently unpredictable: P(E)∝e−(E−Eavg)2/σ2,P(E) \propto e^{-\left(E – E_{avg}\right)^2 / \sigma^2},
where EavgE_{avg} is the average energy, and σ\sigma represents the variability in the tear.
5.2 Implications for the Standard Model
This reinterpretation challenges the Standard Model’s reliance on discrete force carriers. Instead, forces emerge as directional energy flows mediated by the toroidal dynamics of atoms.
6. Mathematical Framework
6.1 Toroidal Energy Distribution
The energy distribution in the torus can be described using spherical coordinates (r,θ,ϕ)(r, \theta, \phi): E(r,θ,ϕ)=E0sin(θ)e−r2/λ2,E(r, \theta, \phi) = E_0 \sin(\theta) e^{-r^2 / \lambda^2},
where E0E_0 is the peak energy, and λ\lambda is the characteristic length scale of the torus.
6.2 Predicting Experimental Outcomes
The torus model predicts distinct outcomes for high-energy collisions, such as those in the LHC. The energy spectrum of the released boson-like events should follow: N(E)=N0e−E/kT,N(E) = N_0 e^{-E / kT},
where kTkT is the thermal energy of the system.
7. Experimental Validation
7.1 Observing Toroidal Patterns
Advanced imaging techniques, such as electron tomography, could reveal toroidal energy distributions in atoms.
7.2 Testing Antimatter Symmetry
High-precision measurements of antimatter’s magnetic moments could confirm the left-hand rule and validate the torus model.
7.3 Collisional Energy Release
Analyzing energy releases in particle collisions for toroidal signatures could provide further evidence.
8. Philosophical Implications
8.1 Redefining Reality
The torus model suggests that reality is inherently directional and dynamic, reshaping our understanding of fundamental physics.
8.2 Bridging Quantum and Relativity
By emphasizing energy flows and geometry, the torus model offers a potential bridge between quantum mechanics and general relativity.
9. Conclusion
The torus atomic model provides a unified framework to address key mysteries in physics, from wave-particle duality to matter-antimatter asymmetry. While challenging established paradigms, it opens new avenues for experimentation and theoretical exploration. The model’s emphasis on directionality, energy flows, and toroidal geometry has the potential to revolutionize our understanding of the universe.
10. References
- Feynman, R. P. (1965). The Feynman Lectures on Physics.
- Einstein, A. (1915). General Theory of Relativity.
- Planck, M. (1900). On the Theory of Energy Quantization.
- Higgs, P. W. (1964). Broken Symmetries and the Masses of Gauge Bosons.
- Dirac, P. A. M. (1930). The Quantum Theory of the Electron.
- Wheeler, J. A. (1968). Geons, Black Holes, and Quantum Foam.